Pg 4. Over the past three years, I have approached these theorems/postulates in different ways.
First attempt: Completed a worksheet and summarized our findings as seen below.
Second attempt:
We completed two flip books with sentence strips that the students had to match the if statement to the then statement and to the diagram.
Third attempt:
Within a large flip book project, we wrote the postulates/theorems out along with each converse.
For the large flip book project, students were to google an image that they could illustrate parallel lines and a transversal on.
INTERESTING: Students brought up the interesting difference between parallel in the real world versus the result of a two dimensional image. For example, one student wanted to use an image of railroad tracks knowing that they were parallel in the real world. However, another student pointed out that when viewed on a two dimensional service the tracks appeared to intersect at the perspective point. Is the picture a valid option? After this discussion, I had students justify their chosen image. How did the idea of parallel apply?
This had some interesting results. I had the students make up some algebra problems, solve and check them, and then exchange with a partner. Some of the results the students would makeup would have infinite solutions, no solution, and result in negative angle measures.
Here are the postulates/theorems and converses.
Out of all that I teach in Geometry, this is one of the hardest concepts to get my students to understand. Proving lines paralell or angles congruent. It took me two years to see the pattern. Maybe next year I will be able to present it better.
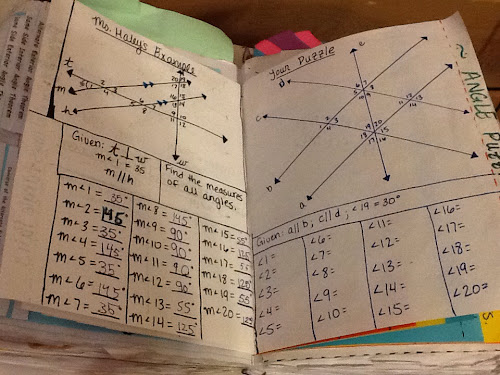
I gave students two angle puzzles to complete. We discussed every detail and justified each strategy used. Next, students were to create their own angle puzzles for homework. The next day, I mixed up each puzzle and passed them out to the class. Great idea, but kind of overwhelming. Students could critique and troubleshoot each others puzzles, but some would use incorrect properties and their answers wouldn't work out. This activity requires quite a bit of time and would probably do better if the students work with a partner.